
Takayuki HOSODA
Dec. 26, 2022

zcbcpw - Analyze Conductor-backed Coplanar Waveguide. Rev.0.82 (Feb. 15, 2023) (c) 2022 Takayuki HOSODA
where
η0 : Impedance of free space 376.730313668(57) Ω
and K(k) is the complete elliptic integral of the first kind.
The above analytical solution is for a negligibly thin conductor thickness, but the conductor thickness used in an actual circuit board has a non-negligible effect. The following formulas are used to compensate the effect of conductor thickness to center strip width w and slot widths g.
Examples of electromagnetic field simulations results (ground plane width W = 12h + 2g + w, upper conductor height H = 8(h + t) Length unit in [ μm ].
Pseudo color visualization of absolute value of the electric field of a cbcpw.
The ratio of the complete elliptic integrals of the first kind K(k) and K(k') can be calculated by the following relation with the ratio of arithmetic-geometric means. The argument k is the elliptic modulus.where agm(1, k) is the arithmetic-geometric mean of 1 and k.

![[Mail]](/~lyuka/images/mail.gif)



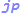 © 2000 Takayuki HOSODA.
© 2000 Takayuki HOSODA.